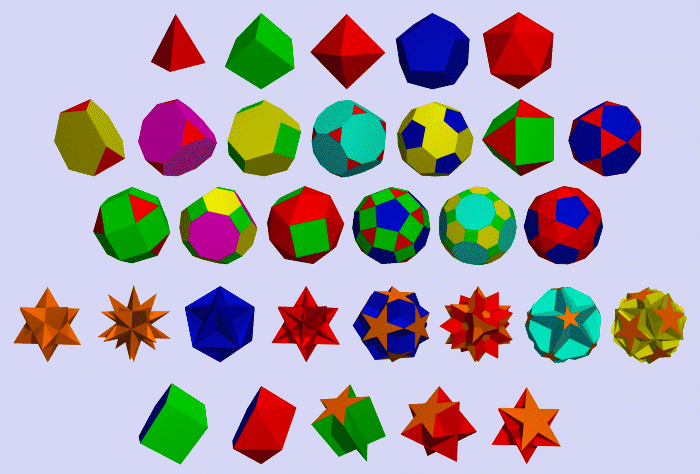
The uniform polyhedra have regular faces and superimposable vertices; they are semi-regular, inscribed in a sphere.
The five regular and the thirteen archimedean solids are convex; among the non convex ones appear the Kepler and Poinsot polyhedra. There are 75 in all; we can add to them the infinite sets of prisms (convex and non convex) and of antiprisms (convex, non convex and crossed). In the list below appear the 5 prisms and antiprisms of order five (2 pentagonal and 3 pentagrammic).
The 75-(5+13)-4=53 non convex and non regular uniform polyhedra are analogous to the archimedean solids but with faces which intersect each other (9 among them have faces which contain the center of the polyhedron); they can be truncated and "snubbed".
Their 53-9=44 finite duals are equifacial and their vertices are regular (as are the Catalan's solids).

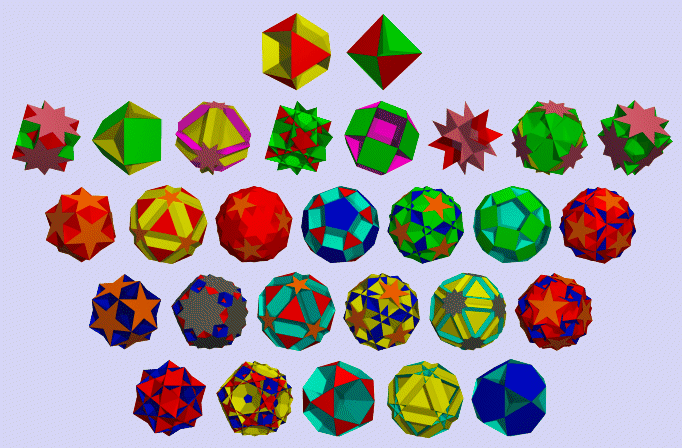
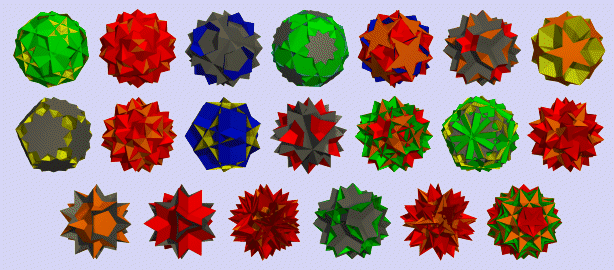
To learn more about the uniform polyhedra, visit the site from where these pictures come.
Only some among them appear as examples on other pages of this site.
| references: |
• uniform polyhedra
• list of uniform polyhedra (Wikipedia) • uniform polyhedra on mathworld (Wolfram Research) by Eric Weisstein • uniform polyhedra on mathcurve.com by Robert Ferréol (in French) • The world of polyhedra by Magnus Wenninger • The 75 uniform compounds of uniform polyhedra site with nice animated pictures and interesting links |
home page
 |
convex polyhedra - non convex polyhedra - interesting polyhedra - related subjects | May 1999 updated 16-10-2004 |