the eight convex deltahedra

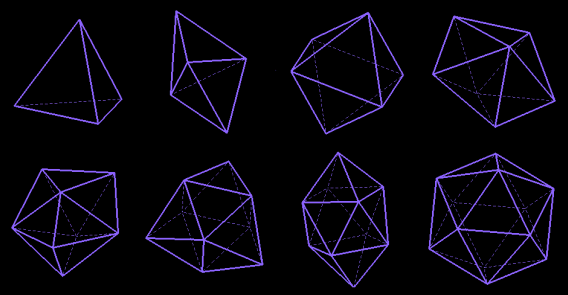
R 1 J 12 R 3 J 13 J 84 J 51 J 17 R 5 deltaèdres

Each drawing provides a link to a popup applet.

All the faces of a deltahedron are equilateral triangles; the number of faces must then be even (two sides of two faces join to form an edge), and the orders of the vertices (numbers of edges meeting at the vertices) can only be 3, 4 or 5.
We know the simplest (the tetrahedron, whose vertices are all of minimum order 3) and the most complex (the icosahedron, whose vertices are all of maximum order 5). Thus only nine deltahedra are possible; we use the notation Df: D4 (tetrahedron), D6, D8 (octahedron), D10... and D20 (icosahedron).
So three are regular, the others are Johnson's polyhedra.
We naturally think of the diamonds which have 2n faces: we get two new deltahedra, D6=J12 and D10=J13, because D8 is known.
If we assemble an antiprism and two pyramids, we get 2n+2n=4n faces, but only D16=J17 is new, because D20 is known and "this D12" has only six faces (some triangles are coplanar).
D14=J51 is the assembling of a triangular prism and three square pyramids.
D12=J84 is deducted from D10 by duplicating one vertex of order 4 to create two new faces.
D12 is also Goldberg's configuration of order 3 (assembling of two deformed dipyramids).
  f     e     v    v3   v4   v5 
4
6
8
10
12
14
16
(18)
20 		6
9
12
15
18
21
24
(27)
30 		4
5
6
7
8
9
10
(11)
12 		4
2
 
 
 
 
 
 
  		 
3
6
5
4
3
2
(1)
  		 
 
 
2
4
6
8
(10)
12

Remark: Concerning D18, we may imagine its characteristics (see the table), but it doesn't exist!
Nevertheless, in Are Your Polyhedra the Same as My Polyhedra?  Branko Grünbaum uses a general definition  for polyhedra which allows points representing distinct vertices to coincide. In this more general context the missing D18 appears geometrically as a regular icosahedron.

home page
French 		convex polyhedra - non convex polyhedra - interesting polyhedra - related subjects April 1999
updated 30-09-2008