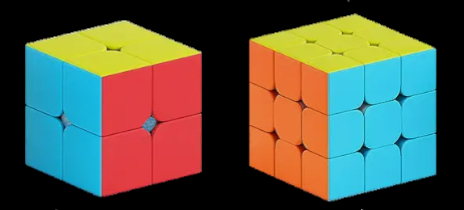

Everyone knows this famous brain teaser, the first copy of which - the Magic Cube - was assembled in 1974 by Hungarian design professor Ernö Rubik. Today there are many versions of different sizes (2x2x2, 4x4x4...) and shapes (tetrahedron, dodecahedron...).
Recently Melinda Green made a puzzle representing in 3D a Rubik's Cube in 4D (hypercube): the 2x2x2x2.
 |
 |
The 2x2x2x2 is made up of sixteen cubes connected by magnets (the two left and right square faces are connected in 4D).
On each cube appear four colors belonging to four pairs (thus eight colors).
Only half a dozen canonical moves are allowed to solve the 2x2x2x2.
| useful references:
(some in French, videos with subtitles translated into English) |
résolution du 2x2x2 tutorial (in French)
solving the 2x2x2 tutorial |
| easy method to solve the 3x3x3 (Le Point hors-série "Logique" pages 18-21 with video demonstration, in French)
solving the 3x3x3 (beginner) https://www.youtube.com/watch?v=uIoYhGVGz6g solving the 3x3x3 (fast 11mn) https://www.youtube.com/watch?v=WIDO5F0myj0 record (2x2x2, 3x3x3, ... 13x13x13) https://www.youtube.com/watch?v=ymIF1sPtcJc | |
| Magic Cube 4D is a program that can simulate Rubik's cubes in 3D and 4D.
solving the 4D Rubik's Cube with simple 3D tricks (1 introduction to Magic Cube 4D) solving the 4D Rubik's Cube with simple 3D tricks (2 building macros) how to solve a 4D Rubik's cube (beginner's method) | |
|
| |
| for the 2x2x2x2: hypercubing
equivalence of virtual movements (on the Magic Cube) and real physical movements | |
| Melinda presents her 2x2x2x2: ordering, assembling, introduction,
canonical moves, scrambling, reorienting
an other presentation of the 2x2x2x2 with comments and explanations (three videos): construction, explanations, solving. solving the physical 2x2x2x2 (beginner's tutorial) https://www.youtube.com/watch?v=hN8VMFSno64 | |
home page
 |
convex polyhedra - non convex polyhedra - interesting polyhedra - related subjects | August 2023 updated 23-08-2023 |